The modification of the natural flow behaviour is one of the fundamental research topics of fluid mechanics. Due to the new unique abilities given by the numerical simulations it is finally possible to get a closer insight into the detailed physics of the flow. Permanently evolving computational capacities enable researchers to tackle the problem of turbulent flows known for their complexity and unpredictability using direct numerical simulations (DNS).
Turbulent skin friction governs the energy losses in various thermo-fluids systems such as airplanes, high-speed rail and motor vehicles, marine vessels or pipeline transportation of fluids. Turbulence control techniques leading to skin friction drag reduction are of great economical and ecological interest because of their potential to achieve efficient energy utilization. Among various turbulence control strategies proposed, reactive control, which is also referred to as feedback control, operates actuators based on the instantaneous flow information obtained by sensors. Thus, it generally enables flexible control of turbulence and offers large energy gain with small control power consumption.
The main feature of a DNS is a complete resolution of temporal and spatial scales in a turbulent flow field. This ability enables reliable application of turbulence control schemes using DNS since the entire multi-scale physics of the manipulation process can be captured. However, in the most cases, the nature of such flow field modification is based on rather unrealistic assumptions directly linked to the properties of DNS. Turbulence control schemes developed using DNS adopt the high spatial and temporal resolution along with the unlimited availability of the flow field information throughout the simulation domain. Correspondingly, the schemes show rather theoretical possibilities of flow control than practically relevant application methods. In the current investigation an attempt is made to examine several representative numerical reactive control schemes with respect to the realistic limitations and establish a link between the numerical flow control schemes and their potential practical application. For this purpose, different limitations are implemented using DNS of a fully developed turbulent channel flow (TCF).
From the engineering point of view, it is more important to be able to modify outer developing turbulent flows, i.e. spatially developing turbulent boundary layers (TBL). Thus, the effect of spatial development of the flow in TBL on the control efficiency is being investinated in order to complete the knowledge base about reactive turbulence control. For this purpose, the local control application is tested in a TBL for clarification of the spatially transient behaviour and its influence on the control efficiency. Based on this knowledge, suggestions about control placement in TBL with a fixed flat plate length are to be made.
The project is being conducted in a close collaboration with the Institute of Industrial Science, The University of Tokyo, Japan (Y. Hasegawa) and the Linné FLOW Cenre, KTH Mechanics, Stockholm, Sweden (Prof. P. Schlatter).
Basic project information
Flow configuration:

• fully developed turbulent channel flow (TCF)
• spatially developing turbulent boundary layer (TBL)

Numerical approach:

• DNS of TCF: finite difference method on a staggered grid with a fractional step method for pressure decoupling
• DNS of TBL: Fourier-Chebyshev solver with streamwise and spanwise periodicity utilizing volume force tripping and fringe region technique

Control techniques:

• uniform blowing/suction
• opposition control
• body force damping
• suboptimal control

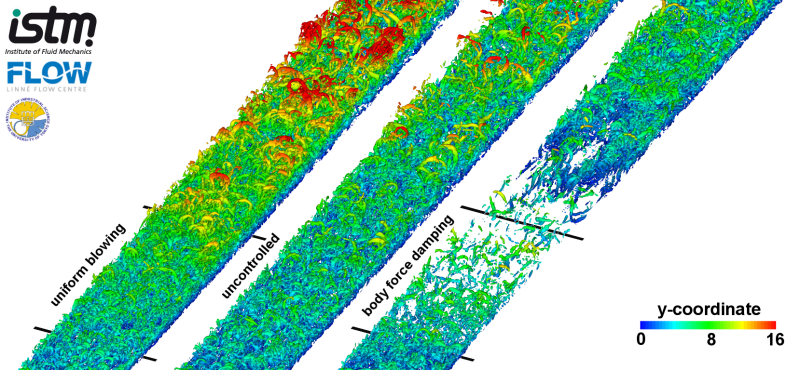
Figure: Visualization of flow structures in uncontrolled and controlled cases represented by isosurfaces of lambda2 criterion (lambda2=-0.005) colored by the wall-normal coordinate